We’ve now talked about vector bundles and locally free sheaves, we’re going to specify to the nicest case: rank 1. We’re generally going to ignore the distinction between the sheaf and the line bundle.
We start by noting an alternative name for locally free sheaves of rank one: invertible sheaves. The reason for this is because given an invertible sheaf 
, we can define 
, the sheaf hom. This turns out to be a locally free sheaf itself, and has rank one. Now, if we look at the sheaf 
(recall that we do this by taking tensor products over open sets, and then sheafifying) we get 
. So in a sense, 
is the inverse for , as their tensor product is not just locally free, but is in fact free.
More is true, in fact. 
is always invertible if both factors are. Now we make note of the following, assuming 
are all line bundles.
.
These isomorphisms should look familiar: they are precisely the axioms for an abelian group! This group is called the Picard group of 
, and denoted 
. Because of this group structure, we will denote 
by 
. We will, however, keep the notation 
for higher rank locally free sheaves.
The fun with the Picard group will REALLY start next week, when I introduce divisors, but there is a bit more we can do before then: like use line bundles to define maps of varieties into projective spaces.
Let be a line bundle. If our variety is defined over the field 
, then 
will be a -vector space. Now, choose a basis 
(if the vector space is trivial, we call the map the empty map to the empty projective space, and so we assume positive dimension 
).
So now we remember that these are not just sheaves, but the sheaves of sections of a geometric vector bundle. We fix a trivialization of the bundle, and on each open set, fix an isomorphism of the fiber with . Then any global section defines a regular function on . So we get a map 
into 
. This map, however, depends on the isomorphisms chosen for the fibers, and the transition functions will multiply it by a scalar as you move from one chart to another, but we can get rid of this dependence by projectivizing. So now we have a rational map 
. It’s only a rational map, because we don’t know in advance if the 
have any common zeros, and the map can’t be defined on those points. And finally, the choice of basis for doesn’t matter, because different bases will give maps that differ only by a linear transformation of the projective space, so we won’t worry about that ambiguity.
So now, we have a rational map for any line bundle. If the map is in fact a morphism, then we call very ample. A line bundle is ample if some tensor power is very ample. There are lots of other characterizations of these line bundles. some of which we’ll encounter.
That’s all for now, next time, we’ll construct a couple of specific vector bundles on nonsingular varieties.
Like this:
Like Loading...
Related
About Charles Siegel
Charles Siegel is currently a postdoc at Kavli IPMU in Japan. He works on the geometry of the moduli space of curves.
Charles Siegel
Jim Stankewicz
Matt DeLand
Note that those axioms are all written in terms of isomorphisms. You’re passing from the collection of invertible sheaves to the collection of isomorphism classes when you define the Picard group. You’re decategorifying!
What you’re implicitly doing is taking the full subcategory of invertible sheaves and showing that it’s a monoidal (and more) subcategory, which is actually groupal (everything has a monoidal inverse up to isomorphism). All the coherence conditions should hold because they do for the whole category of sheaves.
The one thing I’m not sure on (though I think it’s true) is if the monoidal product on sheaves is symmetric, or is it only braided? Either way this subcategory decategorifies to an abelian group.
I, sadly, don’t know enough about braided monoidal categories to answer that.
You’re decategorifying!
Is that a sin?! :-) (Ok, it’s supposed to be a joke!)
Well, what you need is a specific morphism
for each pair of sheaves 
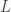and 
, not just the statement that they’re isomorphic. You also need to pick these isomorphisms so that if you have morphisms 
and 
, then the “naturality” condition holds: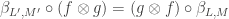 
.
Now the question is whether these isomorphisms are their own inverses. You know that
exists, but is it equal to 
?
If you consider the category of presheaves of modules over your given scheme, then you can define the braiding to be the usual switching of the factors in the tensor product over open sets. This gives a symmetric monoidal structure on presheaves which sheafifies. I’m not really an expert on this sort of stuff, but I think this argument should work… in particular the naturality will be fine.
Right, Greg. I’m pretty sure the obvious direction goes through, but I’m partly fishing around to see if the nontrivial twisting can get you hung up, and partly trying to get people using the categorical-level language to talk about familiar objects.
Well, the biggest reason that I only want to talk about line bundles (and anything else, for that matter) up to isomorphism (or up to embedding, or whatever) is that I’m still learning about categories fibered in groupoids, which is a rather natural structure floating around here. As far as keeping track of isomorphisms, and talking at the categorical (or, dare I say, the higher categorical) level, it’s important, of course, but not what I’m going for with this exposition. Once I have a better grip on stacks, I’m intending to talk about them a little, though not in this series.
I believe the usual definition of a very ample line bundle is that the map from X to P^n is an immersion, not simply that the map is everywhere defined…
And this is what I get for attempting to write a bunch of posts quickly to get a bit ahead. It does need to be an immersion, which is just that for some open subset of
containing the image, the map induces a homeomorphism with the image (which is a closed subset of the open set) and the induced map on sheaves is surjective.
I just thought I’d point out that I think the usual way to define an immersion is to give a factorization as an open immersion followed by a closed immersion, i.e. an open subscheme of a closed subscheme. It is true though that whenever this is the case that you can rewrite in the other order, as a closed immersion then an open one.
However, the converse requires the morphism to be quasi-compact. Working with varieties and good maps though this isn’t really an issue.
Also, keep up the good work ;)
“We fix an isomorphism of each fibre with the field $k$…”: this is not right. You would need to do it in a holomorphic way and you can’t unless yr bundle is trivial (in which case no linear system!). Of course, the bundle is locally trivial so global sections locally give regular functions but these get scaled by the transition functions as I move from one trivialising open set to another. This scaling goes away when I pass to projective space but, in general, there is certainly not a globally defined map into the n+1 dimensional affine space (in fact, if X is compact, any regular function is constant!).
Fran,
You’re right. What I was thinking was more in terms of what you wrote, and the text will be corrected shortly.
Pingback: More on Sheaves | Theories and Theorems